SpringBoot在IDEA中以war打包
本文共 2401 字,大约阅读时间需要 8 分钟。
第一步
在pom.xml中将默认的jar方式改为war:
...homeworkme 0.0.1-SNAPSHOT homeworkme Demo project for Spring Boot war 1.8 ... ...
第二步
排除内置的Tomcat容器(两种方式):
1.排除spring-boot-starter-web中的Tomcat:org.springframework.boot spring-boot-starter-web org.springframework.boot spring-boot-starter-tomcat
2.添加依赖:
org.springframework.boot spring-boot-starter-tomcat provided
第三步
在springBoot启动类的同级目录添加一个继承SpringBootServletInitializer 的类或者启动类继承该类,实现configure方法。
SpringBootServletInitializer源码注释:
Note that a WebApplicationInitializer is only needed if you are building a war file and deploying it. If you prefer to run an embedded web server then you won’t need this at all. 注意,如果您正在构建WAR文件并部署它,则需要WebApplicationInitializer。 如果你喜欢运行一个嵌入式Web服务器,那么你根本不需要这个。
package com.example.homeworkme;import org.springframework.boot.builder.SpringApplicationBuilder;import org.springframework.boot.web.servlet.support.SpringBootServletInitializer;public class ServletInitializer extends SpringBootServletInitializer { public ServletInitializer() { System.out.println("初始化ServletInitializer"); } @Override protected SpringApplicationBuilder configure(SpringApplicationBuilder application) { return application.sources(HomeworkmeApplication.class);//启动类名 }} 第四步
为了防止应用上下文所导致的项目访问资源加载不到的问题,建议pom.xml文件中<build></build>标签下添加<finalName></finalName>标签,并设置maven打包时跳过单元测试:
war包名称 org.apache.maven.plugins maven-surefire-plugin 2.20.1 true org.springframework.boot spring-boot-maven-plugin
第五步
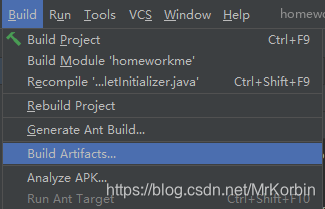
通过maven命令进行打包或者在IDEA顶部菜单栏目中点击Build,再选择Build Artifacts,最后点击build,在项目的target目录下就会生成对应的war包。

最后一步
将生成的war包放到tomcat的webapps目录下,通过ip/项目名访问即可。
注意
当以war包形式打包部署时,使用的是外部Tomcat,这个时候application.yml配置的端口将失效。
转载地址:http://otmg.baihongyu.com/
你可能感兴趣的文章
mysql更新频率_MySQL优化之如何了解SQL的执行频率
查看>>
mysql替换表的字段里面内容
查看>>
MySQL最大建议行数 2000w,靠谱吗?
查看>>
MySQL有哪些锁
查看>>
MySQL服务器安装(Linux)
查看>>
mysql服务器查询慢原因分析方法
查看>>
mysql服务无法启动的问题
查看>>
MySQL杂谈
查看>>
mysql权限
查看>>
mysql条件查询
查看>>
MySQL条件查询
查看>>
MySQL架构与SQL的执行流程_1
查看>>
MySQL架构与SQL的执行流程_2
查看>>
MySQL架构介绍
查看>>
MySQL架构优化
查看>>
mysql架构简介、及linux版的安装
查看>>
MySQL查看数据库相关信息
查看>>
MySQL查看表结构和表中数据
查看>>
MySQL查询优化:LIMIT 1避免全表扫描
查看>>
MySQL查询优化之索引
查看>>